In this article, you will learn how to evaluate a fraction if its limit approaches to infinity and you have an indeterminate form of infinity over infinity. But before learning how to do it, first, we will recall the concepts of limit and l'Hôpital's Rule because both these areas are very closely related to the topic of this article.

What is Limit?
Limit indicates how the function will act if its independent variable, i.e., x approaches to a specific value. Here is a formal definition of the limit:
Let say, a function, f is defined on some open interval that has a number a, except a itself. We can assume that the limit of f(x) as x approaches to a is L, and we can write it mathematically as shown below:
 \lim_ {x\rightarrow a} f(x) = L
\lim_ {x\rightarrow a} f(x) = L
Suppose for every number 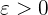 , there is a corresponding number
, there is a corresponding number 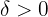 in such as way that:
in such as way that:
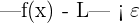
whenever:
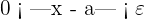
l'Hôpital's Rule
Suppose the function f and g can be differentiated, and the derivative of the function g is g '(x) which is not equal to zero in an interval around a, except a itself. In such as case, one of the following two points can be true:
- The limits of both the functions are zero as x approaches a
- The functions f(x) and g(x) have positive or negative infinite limit as x approaches a
If one of the above discussed points are true, then the limit of the ratio of the functions f and g, i.e., 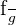 is equal to the limit of the ratio of the differentials of the functions f' and g;, i.e.,
is equal to the limit of the ratio of the differentials of the functions f' and g;, i.e.,  , until the limit persists, or is infinite. We can apply l'Hôpital's Rule on one of the following forms of limit:
, until the limit persists, or is infinite. We can apply l'Hôpital's Rule on one of the following forms of limit:
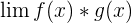 , where f(x) approaches to infinity and g(x) approaches to 0
, where f(x) approaches to infinity and g(x) approaches to 0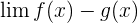 , where both the functions f(x) and g(x) approach infinity
, where both the functions f(x) and g(x) approach infinity , where both the functions f(x) and g(x) approach to infinity
, where both the functions f(x) and g(x) approach to infinity , where both the functions, f(x) and g(x) approach to zero
, where both the functions, f(x) and g(x) approach to zero , where f(x) approaches 1, while g(x) approaches infinity
, where f(x) approaches 1, while g(x) approaches infinity , where both f(x) and g(x) approach to 0
, where both f(x) and g(x) approach to 0 , where f(x) approaches to infinity and g(x) approaches to 0
, where f(x) approaches to infinity and g(x) approaches to 0
In this article, we will specifically discuss how to solve the function whose limit approaches to infinity and we get the indeterminate form 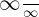 .
.
In the next section, we will evaluate limit functions where both numerator and the denominator are infinite.
Example 1
Evaluate  \lim _ {x \rightarrow \infty} \frac{5x^4 - 2x} {4x^5 + 3x}
\lim _ {x \rightarrow \infty} \frac{5x^4 - 2x} {4x^5 + 3x}
Solution
If we directly apply the limit on the above function, then we will get an indeterminate form of 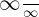 because the numerator
because the numerator  \lim_ {x \rightarrow \infty} 5x^4 - 2x
\lim_ {x \rightarrow \infty} 5x^4 - 2x and the denominator
and the denominator  \lim_ {x \rightarrow \infty} 4x^5 + 3x
\lim_ {x \rightarrow \infty} 4x^5 + 3x both are equal to infinity.
both are equal to infinity.
Here, we will apply l'Hôpital's Rule because it says that the ratio of the functions is equal to the ratio of their derivatives if their limit approaches to infinity. Hence, to apply the l'Hôpital's Rule here, we will take the first derivative of numerator and denominator separately. The first derivative of 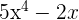 is
is 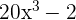 and the derivative of
and the derivative of 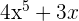 is
is  . Now, write these derivatives in fractional form like this:
. Now, write these derivatives in fractional form like this:
 = \lim_{x \rightarrow \infty} \frac{20x^3 - 2}{20x^4 + 3}
= \lim_{x \rightarrow \infty} \frac{20x^3 - 2}{20x^4 + 3}
If we apply the limit on the above function, we will still get an indeterminate form of 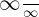 . Hence, now, we will take the second derivative of the above function because it is further differentiable:
. Hence, now, we will take the second derivative of the above function because it is further differentiable:
The second derivative of the numerator 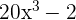 is
is 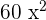 and of the denominator
and of the denominator  is
is  . Write these values in fractional form as shown below:
. Write these values in fractional form as shown below:
 = \lim_{x \rightarrow \infty} \frac{60x^2}{80x^3}
= \lim_{x \rightarrow \infty} \frac{60x^2}{80x^3}
Simplifying it further will give us the following value:
 = \lim_{x \rightarrow \infty} \frac{3}{4x}
= \lim_{x \rightarrow \infty} \frac{3}{4x}
 = \frac{3}{4} \lim_{x \rightarrow \infty} \frac{1}{x}
= \frac{3}{4} \lim_{x \rightarrow \infty} \frac{1}{x}
 = \frac{3}{4} \lim_{x \rightarrow \infty} \frac{1}{\infty}
= \frac{3}{4} \lim_{x \rightarrow \infty} \frac{1}{\infty}
 = \frac{3}{4} \cdot 0
= \frac{3}{4} \cdot 0
 = 0
= 0
Example 2
Evaluate  \lim _ {x \rightarrow \infty} \frac{21x} {10x - 9}
\lim _ {x \rightarrow \infty} \frac{21x} {10x - 9}
Solution
If we directly apply the limit on the above function, then we will get an indeterminate form of 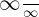 because the numerator
because the numerator  \lim_ {x \rightarrow \infty} 21x
\lim_ {x \rightarrow \infty} 21x and the denominator
and the denominator  \lim_ {x \rightarrow \infty} 10x - 9
\lim_ {x \rightarrow \infty} 10x - 9 both are equal to infinity.
both are equal to infinity.
Here, we will apply l'Hôpital's Rule because it says that the ratio of the functions is equal to the ratio of their derivatives if their limit approaches to infinity. Hence, to apply the l'Hôpital's Rule here, we will take the first derivative of numerator and denominator separately. The first derivative of 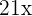 is
is  and the derivative of
and the derivative of 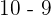 is
is  . Now, write these derivatives in fractional form like this:
. Now, write these derivatives in fractional form like this:
 = \lim_{x \rightarrow \infty} \frac{21}{10}
= \lim_{x \rightarrow \infty} \frac{21}{10}
 = 2.1
= 2.1
Example 3
Evaluate  \lim _ {x \rightarrow \infty} \frac{3x^3 + 2x + 1} {5x^3 + 8x + 10}
\lim _ {x \rightarrow \infty} \frac{3x^3 + 2x + 1} {5x^3 + 8x + 10}
Solution
If we directly apply the limit on the above function, then we will get an indeterminate form of 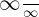 because the numerator
because the numerator  \lim_ {x \rightarrow \infty} 3x^3 + 2x + 1
\lim_ {x \rightarrow \infty} 3x^3 + 2x + 1 and the denominator
and the denominator  \lim_ {x \rightarrow \infty} 5x^3 + 8x + 10
\lim_ {x \rightarrow \infty} 5x^3 + 8x + 10 both are equal to infinity.
both are equal to infinity.
Here, we will apply l'Hôpital's Rule because it says that the ratio of the functions is equal to the ratio of their derivatives if their limit approaches to infinity. Hence, to apply the l'Hôpital's Rule here, we will take the first derivative of numerator and denominator separately. The first derivative of 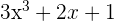 is
is 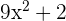 and the derivative of
and the derivative of 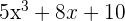 is
is  . Now, write these derivatives in fractional form like this:
. Now, write these derivatives in fractional form like this:
 = \lim_{x \rightarrow \infty} \frac{9x^2 + 2}{15x^2 + 8}
= \lim_{x \rightarrow \infty} \frac{9x^2 + 2}{15x^2 + 8}
If we apply the limit on the above function, we will still get an indeterminate form of 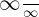 . Hence, we will take the second derivative of the above function because it is further differentiable:
. Hence, we will take the second derivative of the above function because it is further differentiable:
The second derivative of the numerator 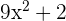 is
is  and of the denominator
and of the denominator  is
is 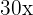 . Write these values in fractional form as shown below:
. Write these values in fractional form as shown below:
 = \lim_{x \rightarrow \infty} \frac{18x}{30x^3}
= \lim_{x \rightarrow \infty} \frac{18x}{30x^3}
Simplifying it further will give us the following value:
 = \lim_{x \rightarrow \infty} \frac{3x}{5x}
= \lim_{x \rightarrow \infty} \frac{3x}{5x}
 = \frac{3}{5}
= \frac{3}{5}
Example 4
Evaluate  \lim _ {x \rightarrow \infty} \frac{ln x} {e^x + 3}
\lim _ {x \rightarrow \infty} \frac{ln x} {e^x + 3}
Solution
If we directly apply the limit on the above function, then we will get an indeterminate form of 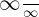 because the numerator
because the numerator  \lim_ {x \rightarrow \infty} ln x
\lim_ {x \rightarrow \infty} ln x and the denominator
and the denominator  \lim_ {x \rightarrow \infty} e^x + 3
\lim_ {x \rightarrow \infty} e^x + 3 both are equal to infinity.
both are equal to infinity.
Here, we will apply l'Hôpital's Rule because it says that the ratio of the functions is equal to the ratio of their derivatives if their limit approaches to infinity. Hence, to apply the l'Hôpital's Rule here, we will take the first derivative of numerator and denominator separately. The first derivative of  is
is  and the derivative of
and the derivative of 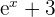 is
is 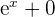 . Now, write these derivatives in fractional form like this:
. Now, write these derivatives in fractional form like this:
 = \lim_{x \rightarrow \infty} \frac{\frac{1}{x}}{e^x}
= \lim_{x \rightarrow \infty} \frac{\frac{1}{x}}{e^x}
 = \frac{0}{\infty} = 0
= \frac{0}{\infty} = 0
Example 5
Evaluate  \lim _ {x \rightarrow \infty} \frac{ln x} {\sqrt{x} + 5}
\lim _ {x \rightarrow \infty} \frac{ln x} {\sqrt{x} + 5}
Solution
If we directly apply the limit on the above function, then we will get an indeterminate form of 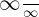 because the numerator
because the numerator  \lim_ {x \rightarrow \infty} ln x
\lim_ {x \rightarrow \infty} ln x and the denominator
and the denominator  \lim_ {x \rightarrow \infty} \sqrt{x} + 5
\lim_ {x \rightarrow \infty} \sqrt{x} + 5 both are equal to infinity.
both are equal to infinity.
Here, we will apply l'Hôpital's Rule because it says that the ratio of the functions is equal to the ratio of their derivatives if their limit approaches to infinity. Hence, to apply the l'Hôpital's Rule here, we will take the first derivative of numerator and denominator separately. The first derivative of  is
is  and the derivative of
and the derivative of 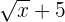 is
is 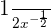 . Now, write these derivatives in fractional form like this:
. Now, write these derivatives in fractional form like this:
 = \lim_{x \rightarrow \infty} \frac{\frac{1}{x}}{\frac{1}{2} x^{-\frac{1}{2}}}
= \lim_{x \rightarrow \infty} \frac{\frac{1}{x}}{\frac{1}{2} x^{-\frac{1}{2}}}
 = 2\lim_{x \rightarrow \infty} \frac{\frac{1}{x}}{ \frac{1}{\sqrt{x}}}
= 2\lim_{x \rightarrow \infty} \frac{\frac{1}{x}}{ \frac{1}{\sqrt{x}}}
 = 2\lim_{x \rightarrow \infty} \frac{\sqrt{x}} {x}
= 2\lim_{x \rightarrow \infty} \frac{\sqrt{x}} {x}
 = 2\lim_{x \rightarrow \infty} \frac{1} {\sqrt{x}}
= 2\lim_{x \rightarrow \infty} \frac{1} {\sqrt{x}}













Correct equals infinity equals 16 but not true. It’s it’s six it’s infinity.
∞ = -1/12
By Sriniwas aramanujan
And this, my friends, is why humans will be conquered by AI… so many logic holes it’s… well, infinite. lol
Is 0^infinity ( zero to the power infinity) indeterminate form? How?
Didn’t Cantor proved that there are a group of infinities (the Aleph zero & Aleph one sets, for example)? And these are grouped around the concept of infinite to the power infinity, if I remember correctly .
Exercise 3
I think it is discontinuous at 0
exercise 2 : the function is not defined for x= 0.
exercise 1 q 5 The function has a jump discontinuity at x = 1, should be x=0.